Lecture 13: Statistical mechanics of an ideal gas

Note

It is when they take a deeper dive into statistical mechanics and start to see the light that physicists realize they are part of a special club. – V. Meunier

Warning

This lecture corresponds to Chapter 21 of the textbook.

Summary

Attention

Since Lecture 12: The partition function, we realized that the knowledge of the partition function is all we need to extract all the thermodynamical properties of a given system (at equilibrium) That’s a big deal!

We are able to calculate the partition function for fairly simple problems as we saw in the previous lecture. We even came up with a scheme to combine partition functions corresponding to different energy contributions, so long as those contributions are independent.

Most of the rest of this course will be devoted to calculating the partition functions for various scenarios. The good news is that this knowledge will suffice to derive all we want to know about the systems… the bad news is that it can be exceedingly difficult to calculate partition functions!

In this lecture, we will consider the case of a gas confined to a cubic box of length $L$ . We will consider that the gas molecules do not interact with one another and that they move freely inside the box. We have already encountered this scenario: this is the ideal gas!

How do we formalize the confinement of a single gas molecule in a box? We remember from elementary quantum mechanics that a particle confined to a box must see its wave-function vanish at the boundary. It follows that the wave-function must be (in 3D):

$\psi(x, y, z)=\left(\frac{2}{L}\right)^{3 / 2} \sin \left(k_{x} x\right) \sin \left(k_{y} y\right) \sin \left(k_{z} z\right),$

where the only acceptable momenta (to ensure proper boundary conditions) must be

$k_{x}=\frac{n_{x} \pi}{L}, \quad k_{y}=\frac{n_{y} \pi}{L}, \quad k_{z}=\frac{n_{z} \pi}{L},$

where $n_{x}$ , $n_{y}$ , and $n_{z}$ are positive integers.

The total energy of each state is simply due to kinetic contributions:

$E_{n_{x}, n_{y} n_{z}}=\frac{h^{2}}{8 m}\left(\frac{n_{x}^{2}}{L^{2}}+\frac{n_{y}^{2}}{L^{2}}+\frac{n_{z}^{2}}{L^{2}}\right).$

Because of the constraint on the momentum, we see that each state occupies a volume $(\pi / L)^{3}$ in momentum space.

We spent quite a bit of time (see screencast) to calculate the density of states. The density of states is the number of states with momentum between $k$ and $k+dk$ :

$g(k) d k=\frac{V k^{2} d k}{2 \pi^{2}}.$

We note that all those states have the same energy.

Using this equation, we can evaluate the partition function:

$Z_{1}=\int_{0}^{\infty} e ^{-\beta E(k)} g(k) d k \text { with } E(k)=\frac{\hbar^{2} k^{2}}{2 m}$

or

$Z_{1}=\frac{V \hbar}{3}\left(\frac{m k_{ B } T}{2 \pi}\right)^{3 / 2}.$

We use the subscript (1) to make it clear that this is the partition function of a single particle.

Now, we are ready to move to a collection of independent gas molecules. Unfortunately, there is a difficulty we did not anticipate! The gas molecules are indistinguishable and, as a result, one cannot just write that the partition function of $N$ independent gas molecules is $(Z_1)^N$ ! This difficult is discussed in details in the blue box below. To address this issue, we make the approximation that as long as the number of available states is much smaller than the number of particles, we have:

$Z_{N}=\frac{\left(Z_{1}\right)^{N}}{N !}=\frac{1}{N !}\left(\frac{V}{\lambda_{ th }^{3}}\right)^{N}.$

Just like we did in the previous lecture, we can now calculate thermodynamic potentials using the usual formula involving the partition function. We rediscover a number of results we found before, such as the equipartition theorem and the ideal gas equation. In addition, we also have an expression for the entropy (also known as the Sackur-Tetrode equation):

$S=N k_{ B }\left[\frac{5}{2}-\ln \left(n \lambda_{ th }^{3}\right)\right].$

This equation allows use to revisit the problem of Joule Expansion to confirm what we discussed before. Furthermore, this allows us to stress the importance of distinguishability in thermodynamics and, thus, resolve the apparent paradox that mixing two identical gas yields no change in entropy (Gibbs).

We conclude this lecture with a discussion of heat capacity of a diatomic gas since its partition function can be obtained by combining 3 translations, 2 rotations and 2 degrees of freedom related to vibration.

Note

Indistinguishable particles

One very critical aspect of modern physics is understanding the consequences of indistinguishability between particles. This concept has profound consequences on the statistical mechanics of identical particles. To illustrate this, let’s consider the figure below for a specific case (note: in the screencast, we looked a 2 particles in a 2-state system; here we will look at 2 particles in a 3-state system).

First, consider the figure below where we have 2 distinguishable particles (marked by the $\circ$ and $\bullet$ symbols). It is clear we have 9 possibilities: 3 possibilities for $\circ$ combined with 3 possibilities for $\bullet$ . In this case, if we know the partition function for each individual particle in the 3-state system, we know that the combined partition function will be:

$Z=Z_{\circ}Z_{\bullet}.$

Second, imagine that both particles are exactly the same (in other words, they are indistinguishable). Naively, we would end up with the situation depicted below.

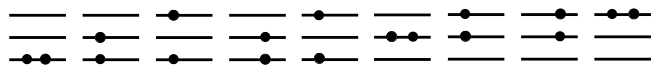
This picture is obviously wrong since we double counted a number of situations. In fact, we double counted all situations where the particles occupy different levels.

The correct picture is, in fact:

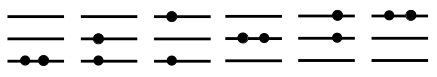
So we see there is a major difficulty to combine the partition functions of independent particles when they are indistinguishable!

The approximation that we will use goes as follows. We start by realizing that when the number of available states is much larger than the number of particles, the majority of the many-body states will correspond to single occupancies. We know that single-occupancy states are over-counted (as we just described) by a factor $N!$ . This leads to the following approximation:

$Z_N=\frac{Z_{1}^N}{N!}.$

The question is: when is the number of available states large enough? It appears that the criteria is related to the concept of quantum concentration. If the density of particles in a system is much smaller than the quantum concentration, the approximation is quite good.

Key Definitions

Note

Quantum concentration:: $n_{ Q }=\frac{1}{\hbar^{3}}\left(\frac{m k_{ B } T}{2 \pi}\right)^{3 / 2}$
Thermal wavelength:: $\lambda_{ th }=n_{ Q }^{-1 / 3}=\frac{h}{\sqrt{2 \pi m k_{ B } T}}$
Indistinguishable particles:: Identical particles exhibit critically different statistical behaviors compared to distinguishable ones. Gibbs pushed this point to resolve the mixing paradox in the Joule expansion experiment.

A full list of terms, including the ones provided here, can be found in the Index.

Learning Material

Copy of Slides

The slides for Lecture 13 are available in pdf format here: pdf

Screencast

Test your knowledge

Statistical mechanics of a gas is built upon the fact we impose the particles to be confined in a given volume. The fact that a series of discrete states is induced by such a confinement stems from a quantum mechanical treatment.
1. True
2. False
3. It depends whether or not we get another deadline extension in the class
You are asked to calculate the partition function of a gas of hydrogen atoms. You know it is a difficult task and you decide to ask classmates to work out collectively a viable solution. Among the claims below, which one is correct?
1. The problem is as long as QP2 professor’s beard and there is little hope to finish it on time.
2. This is not a good idea as the partition function depends on many different properties, including individual energylevels and it is difficult to share this work among students.
3. This is a good idea: you can take advantage of the fact that each mode of a partition function contributes an individual partition function that is just a factor used to build the entire partition function. Of course you know you can’t really build the full partition function but the more factors you include, the closer you get to the right answer.
4. This is a good idea: you can take advantage of the fact that each mode of a partition function contributes an individual partition function that is just a factor used to build the entire partition function. This way you only need two factors to build the exact partition function: one for the fact this is a gas and one for the fact you know how individual hydrogen atoms’s energy levels vary with the principal quantum number $n$ .
5. None of the other answers is correct.
Thinking about the statistical mechanics of an ideal gas…
1. An ideal gas is a purely classical system where no quantum mechanical property is accounted for.
2. An ideal gas is a purely classical system where no relativistic effect can be accounted for.
3. An ideal gas can be described within a quantum mechanical framework; for instance by imposing each individual state to occupy a specific volume in wave-vector space.
4. An ideal gas is a gas where the interactions are weak and can be described by Hooke’s law. This, in turn, can be described by the quantum mechanical properties of harmonic oscillators.
5. None of the other claims is correct.
When building the partition function of a collection of ideal gas molecules that are indistinguishable…
1. The partition function is simply the product of the partition functions of individual molecules since they do not interact.
2. The partition function is simply the sum of the partition functions of individual molecules since they do not interact.
3. The partition function is simply the product of the partition functions of individual molecules, divided by $N!$ to get the exact partition function of the gas.
4. The partition function is simply the product of the partition functions of individual molecules, divided by $N!$ to get an approximate partition function of the gas.
You are asked to calculate the partition function of an ideal gas and then obtain its internal energy.
1. You cannot do this since there is no way to know the partition function.
2. An ideal gas is, by definition, a low-density ensemble of gas molecules, therefore using the $N!$ normalization is accurate when evaluating the partition function. Incidentally, doing this, you find that the internal energy matches the result of the equipartition theorem.
3. An ideal gas is, by definition, a low-density ensemble of gas molecules, therefore the normalization using $N!$ is accurate. Incidentally, doing this, you do not find the result of the equipartition theorem since the particles are indistinguishable.
4. None of the other claims is correct.

Hint

Find the answer keys on this page: Answers to selected test your knowledge questions. Don’t cheat! Try solving the problems on your own first!

Homework Assignment

Solve the following problems from the textbook: